The recreational visit of the “Stairs” bar with accompanying tasting of different cocktails which form a creativity stairs – classic, with twist or signature, lead to the discussion on recreational mathematics. The Penrose stairs, impossible objects as well as seemingly impossible aperiodic coverage of infinite surface with tiles, which once proved, culminated in discovery of quasi-crystals and change of the definition of the crystal itself.
What?
Stairs bar
Prices?
12-15 Euro/cocktail
Introduction
The “Stairs” bar is located in the Uhlandstraße in Berlin-Wilmersdorf. The entrance is hidden. In fact we had to ask for location and still missed it. After ringing the doorbell we were let in and landed in the part of the location, which probably gave the bar its name. The staircase leads from the entrance up to the bar. An impressing detail is that you go up until you can look down onto location and then descend into the first room, where the counter is located.
Of course the designer used bars name as inspiration and therefore above the bar the shelf with the spirits takes the form of the stairs and also patterns on the walls and the chandelier are inspired by the staircases.
A similar principle can be found in other areas in the bar. In the menu and in the concept itself. Each page of the menu offers an ascending in creativity, as you can choose between a classical cocktail, the same cocktail with a twist and a house specialty. The atmosphere and the quality of the drinks as well as the creativity make it worth the price. We tried Aviation, Violett Fizz, Womanhattan, Aged Rob Roy, Clover Club and Spit and Stars. The Aviation is a classic, which is hard to balance. The original recipe, invented 1916, as served in “Stairs” contains Creme de Violette, which was hard to get in Europe and USA for decades and therefore there is a later and much sourer version without Creme de Violette and more Maraschino liqueur. I love sour cocktails, but in this case I fashion the elusive taste of Creme de Violette as well as the vanishing blue colour of the cocktail. The Aviation with a twist is Violett Fizz, which replaces Maraschino with sugar, Aqua Faba and soda. It gives the cocktail additional sparkling feeling, but makes it less strong. It is refreshing, has the violet taste and will please any Fizz lover. Aged Rob Roy, Clover Club and Spit and Stars are all cocktails with a twist. Clover Club as a variation of a White Lady was pleasant but felt featureless. Spit and Stars is twist on Sand and Blood, which was heavy on taste and alcohol, but thrilling and fitting the ambience of the bar. I enjoyed the Aged Rob Roy, which is a version of Rob Roy aged for three months in a port barrel. The process gives this cocktail a distinguishable note in taste and I recommend it to anyone, who likes the barrel-aged drinks.
The extraordinary about “Stairs” is the sustainability, which might lead mixologists stepwise into waste free future. The chef of the bar – Konstantin Hennrich, takes care that the drinks are served in highest quality, but as sustainable as possible. Instead of lemons and limes, lemon- and lime water are used, which can be made from peels and have no difference in taste. The drinks are served with glass straws and the decoration is reprocessed into a tea later. To give you the possibility to make a contribution to sustainable mixing the recipe for lime (lemon) water invented by Konstantin is posted below.
The lime (lemon) water recipe
- 940g filtered water
- 40 (60) g citric acid
- 20 (0) g malic acid
- 8g fructose
- 6g glucose
- 10g lime (lemon) peels
- 0.04g succinic acid
Discussion
The stairs have a significant meaning as a metaphor. Usually as a passage – either positive (ascending) or negative (descending). Also the shape might play a role – straight stands for easy passage as spirals, angles, etc. mean trouble on your way. This metaphor can be surprisingly extended to the world of mathematics. If we take a look at the Penrose stairs we will see a never-ending passage. Even though our brain realizes that it deals with optical illusion it fails to break it.

The pioneers of the impossible figures were M. Duchamp in 1916 and O. Reutersvärd in 1934, but it was the impossible cube designed by C. Escher for Belvedere, which inspired R. Penrose and his father L. Penrose to design a number of impossible objects (British Journal of Psychology, 1958; Sage Encyclopedia of Perception, 2010). This specific objects were part of what R. Penrose called a recreational mathematics. Another seemingly impossible pattern – the Penrose tiles – was discovered by him as he was performing recreation in a similar way.
The Penrose tiles can cover an infinite surface forming a pattern, which never repeats itself. The mathematical problem itself falls under recreational mathematics but unexpectedly has consequences in different scientific areas. The problem was already formulated by H. Wang, as he proposed 20426 types of colored dominos (prototiles) and assumed that if this problem (covering an infinite area with dominos) is noncomputable then an aperiodic coverage exists. Wang assumed that the problem should be computable and therefore no aperiodic set of tiles exists. With this assumption he also connected computability and computer algorithms with aperiodic tiles (The Bell System Technical Journal, 1961).
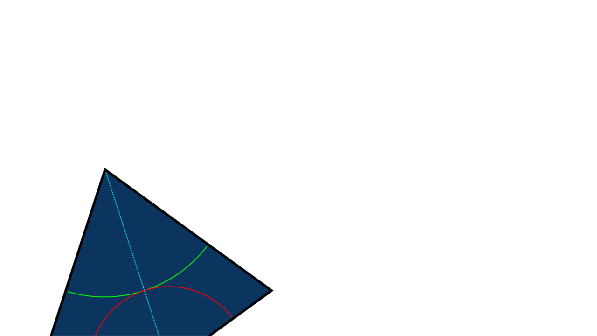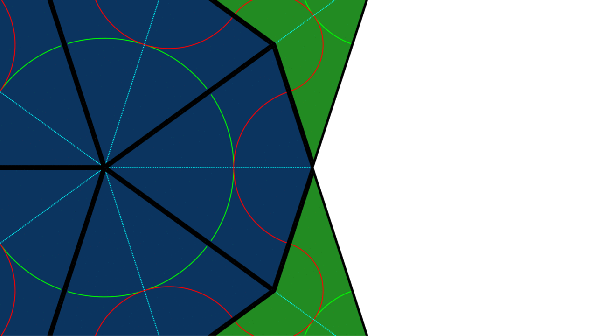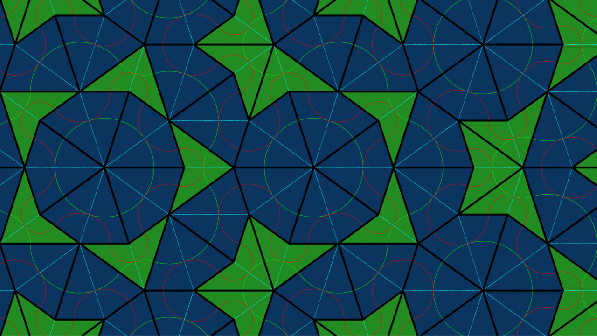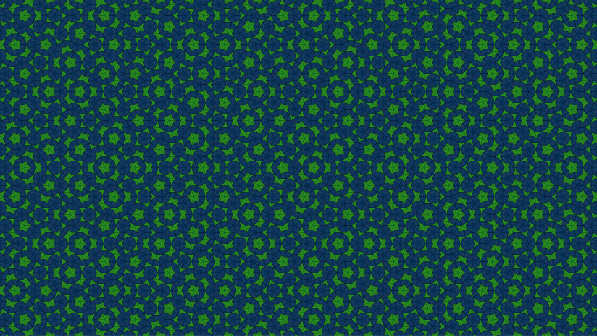
Wang’s student Robert Berger showed that Wang’s assumption was wrong and the tiling is non-computable as well as slashed the number of necessary tiles to 104 (Memoirs of the American Mathematical Society, 1966). R. Robinson simplified the proof for this problem and lowered the number of prototiles to just 6 (Inventiones matematicae, 1971). R. Penrose started out with 6 types of tiles, but could reduce them to just two. Using these two types it is possible to build a non-periodic tiling, which would cover infinite plane without repeating itself (Eureka, 1978).
If we would take square tiles – we can cover any surface. However, if we cut out part of the tiles, move them in any direction by whole number of tiles and replace tiles there, we would see no difference. The Penrose tiles are different – in some cases they can be mirrored or turned around a center, but if you move them the pattern is broken.
The patterns are beautiful of course and for mathematicians it can be recreational to solve this kind of problems, but surprisingly it is not the end of the story. First of all based on this fun new structures were found, which were named “quasi-crystals” (Physical Review Letters, 1984) . They follow the same rules as Penrose tiling rules and form a structure. However, this structure does not repeat itself, like in usual crystals. Crystals are formed if a periodic structure is given. Quasi-crystals form solid matter, but with aperiodic structure only (like Penrose tiles). As a consequence if an atom is shifted in this structure the whole structure has to rearrange. This speaks for simultaneous forming of matter as opposed to e.g. growing layer by layer. The consequences are tremendous as this new kind of crystals has different and novel features, such as very low friction, which makes them ideal as e.g. coatings for razor blades. In 1992 the International Union of Crystallography had to establish a new committee to redefine the ‘crystal’ itself. The tiling resulted in the astonishing ‘unnatural’ structures, but it was also discovered that in islamic culture this particular pattern were known and used for adornment of mosques (Science, 2006).
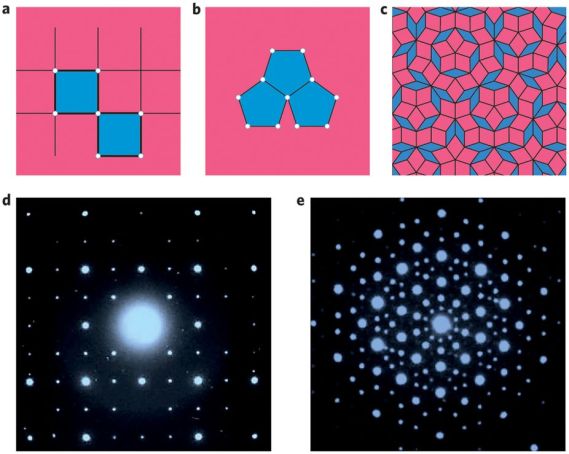

We made a recreational journey from the stairs in the bar to the strange pattern in mathematics, nature and ancient ornaments, but we should not forget Prof. Wang. His proposition that Penrose tiling is not computable might give us a thin edge for distinguishing between conscious and unconscious, as a machine by definition uses algorithms, but human conscious brain does not. And therefore it is possible that by proving that noncomputable patterns exist, which were discovered by humans by now, we also prove that machines cannot gain a consciousness (Toward a Science of Consciousness – The First Tucson Discussions and Debates, 1996).
Summary
Recreation in any form is an important part of human culture. It does relax but also stimulates creativity and search of new ways and new impressions. This is valid for any bar, but especially for “Stairs” bar, where the range of the themes covers mixology, design, mood and sustainability. However, any kind of recreation can lead to a significant discoveries, as shown by R. Penrose with Penrose stairs, impossible objects and Penrose tiling. His recreational discoveries demonstrate vividly how much recreation and lively discussion can be worth. So during your next recreational visit of a bar be prepared to change the world, eventually!
References
- L. S. Penrose and R. Penrose, Impossible Objects: A Special Type of Visual Illusion. British Journal of Psychology, 41(9): 31-33 (1958)
- M. Macpherson, Impossible Figures. Sage Encyclopedia of Perception (2010)
- H.Wang, Proving theorems by pattern Recognition – II. The Bell System Technical Journal, 40(1):1-41 (1961)
- R. Berger, The undecidability of the domino problem. Memoirs of the American Mathematical Society, 66 (1966)
- R. M. Robinson, Undecidability and nonperiodicity for tilings of the plane. Inventiones matematicae, 12: 177-209 (1971)
- R. Penrose, Pentaplexity: A Class of Non-Periodic Tilings of the Plane. Eureka, 39: 32-37 (1978)
- D. Shechtman, I. Blech, D. Gratias and J. W. Cahn, Metallic Phase with Long-Range Orientational Order and No Translational Symmetry. Physical Review Letters, 53 (20): 1951-1953 (1984)
- D. Levine and P. J. Steinhardt, Quasicrystals: A New Class of Ordered Structure. Physical Review Letters, 53(20): 2477-2480 (1984)
- P. J. Lu and P. J. Steinhardt, Decagonal and Quasi-Crystalline Tilings in Medieval Islamic Architecture. Science, 315(5815):1106-1110 (2006)
-
Svetlana V. Boriskina Making invisible materials. Nature Photonics volume 9, pages 422–424 (2015)
- S. Hameroff and R. Penrose, Orchestrated Objective Reduction of Quantum Coherence in Brain Microtubules: The “Orch OR” Model for Consciousness. Toward a Science of Consciousness – The First Tucson Discussions and Debates, 506-540 (1996)





